Mastering The Standard Form Polynomial Expression: A Deep Dive

Polynomials are like the superheroes of algebra, and understanding the standard form polynomial expression is like unlocking their secret powers. Whether you're a high school student diving into math or an adult brushing up on your algebra skills, knowing how to work with polynomials is essential. This article will take you on a journey through the world of polynomials, focusing on their standard form, why it matters, and how to master it effortlessly.

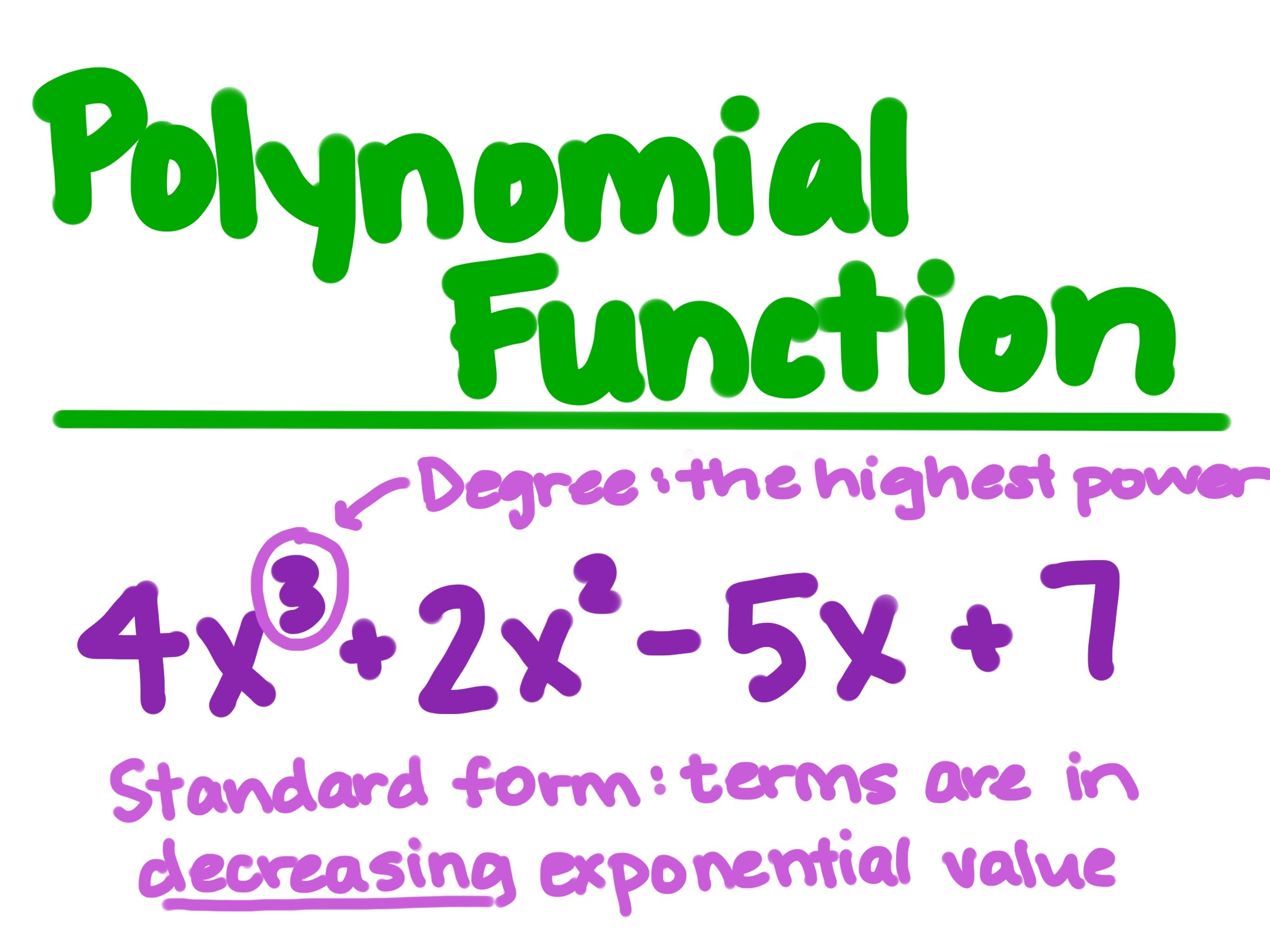
Now, you might be wondering, "What exactly is a standard form polynomial expression?" Well, it's like organizing your closet—everything has its place, and things make more sense when they're in order. In algebra, putting a polynomial into standard form means arranging its terms in descending order of their degrees. This simple yet powerful concept opens doors to solving equations, graphing functions, and even real-world applications like engineering and physics.

But don't worry, we're not just throwing numbers at you. This guide is packed with examples, tips, and tricks to help you grasp the concept effortlessly. Whether you're here to ace your next math test or simply want to expand your knowledge, we've got you covered. Let's get started!

Read also:

How Tall Is Laura Ingraham In Feet And Inches The Surprising Truth About Her Height

Before we dive deeper, here's a quick roadmap to help you navigate:

• What is a Standard Form Polynomial Expression?
• Why is Standard Form Important?
• A Brief Biography of Polynomials
• Breaking Down the Components of Polynomials
• How to Convert Polynomials to Standard Form
• Practical Examples of Standard Form
• Common Mistakes to Avoid
• Real-World Applications of Standard Form
• Tips for Students Struggling with Polynomials
• Wrapping It Up

What is a Standard Form Polynomial Expression?

Alright, let's break it down. A polynomial is an algebraic expression made up of variables, coefficients, and exponents. Think of it as a recipe where you mix different ingredients to create something amazing. The standard form polynomial expression is all about organizing these ingredients in a specific way.

In standard form, the terms of a polynomial are arranged from the highest degree to the lowest. For example, if you have the polynomial 3x² + 5x - 7, it's already in standard form because the term with the highest degree (3x²) comes first, followed by the next highest (5x), and finally the constant term (-7).

Why does this matter? Well, just like how a well-organized recipe makes cooking easier, putting polynomials in standard form simplifies solving equations, graphing, and analyzing functions. It's like giving your math problems a neat haircut—everything looks cleaner and more manageable.

Why is Standard Form Important?

Standard form isn't just about aesthetics; it's a practical tool that makes algebraic operations smoother. Here's why:

• Clarity: When polynomials are in standard form, it's easier to identify the degree of the polynomial, which is the highest power of the variable.
• Consistency: Standard form ensures that everyone is on the same page when working with polynomials, reducing confusion and errors.
• Efficiency: Operations like addition, subtraction, and multiplication become more straightforward when polynomials are organized in standard form.

Imagine trying to solve a complex equation with terms scattered all over the place. Sounds frustrating, right? Standard form eliminates that frustration by bringing order to chaos.

Read also:

Commuter Rail Meaning Your Ultimate Guide To Understanding Commuter Rails

A Brief Biography of Polynomials

Let's take a step back and talk about where polynomials come from. Polynomials have been around for centuries, with roots tracing back to ancient civilizations like the Babylonians and Greeks. These early mathematicians used polynomial-like expressions to solve practical problems like measuring land and predicting astronomical events.

Fast forward to the modern era, and polynomials are everywhere—in science, engineering, economics, and even everyday life. They're the building blocks of calculus, the backbone of computer algorithms, and the secret sauce behind many technological advancements.

Here's a quick snapshot of some famous polynomial pioneers:

Name	Contribution	Year
Euclid	Early work on algebraic expressions	300 BC
Al-Khwarizmi	Introduced systematic solutions to polynomial equations	820 AD
René Descartes	Developed coordinate geometry, paving the way for polynomial graphs	1637

Breaking Down the Components of Polynomials

Now that we know what polynomials are and why standard form matters, let's dissect their components:

• Variables: These are the letters in a polynomial, like x or y. They represent unknown values.
• Coefficients: These are the numbers multiplied by the variables, like the 3 in 3x².
• Exponents: These tell you how many times a variable is multiplied by itself, like the ² in x².
• Constants: These are standalone numbers without variables, like -7 in 3x² + 5x - 7.

Understanding these components is crucial because they determine the structure and behavior of a polynomial. For example, the degree of a polynomial (the highest exponent) affects its shape when graphed.

How to Convert Polynomials to Standard Form

Converting a polynomial to standard form is simpler than you think. Follow these steps:

1. Identify all the terms in the polynomial.
2. Arrange the terms in descending order of their degrees.
3. Simplify if necessary by combining like terms.

Let's try an example:

Suppose you have the polynomial 7x - 2x³ + 4 + 5x². To put it in standard form:

1. Identify the terms: -2x³, 5x², 7x, 4.
2. Arrange in descending order: -2x³ + 5x² + 7x + 4.
3. Simplify (if needed): In this case, no further simplification is required.

Voilà! You now have a polynomial in standard form.

Practical Examples of Standard Form

Let's look at a few more examples to solidify your understanding:

• 4x² + 3x - 8 is already in standard form.
• 9 + 2x - 6x² becomes -6x² + 2x + 9 in standard form.
• x³ - 4x + 5x² - 2 becomes x³ + 5x² - 4x - 2 in standard form.

Each example demonstrates how arranging terms in descending order brings clarity and structure to the polynomial.

Common Mistakes to Avoid

Even the best mathematicians make mistakes sometimes. Here are a few pitfalls to watch out for:

• Forgetting to arrange terms in descending order: Always double-check that your polynomial is in standard form.
• Missing like terms: Be sure to combine terms with the same variable and exponent.
• Ignoring constants: Constants are important too! Don't leave them out.

By avoiding these common errors, you'll save yourself a lot of headaches and ensure your polynomials are in tip-top shape.

Real-World Applications of Standard Form

Polynomials aren't just abstract concepts confined to math textbooks. They have real-world applications that touch almost every aspect of modern life:

• Engineering: Polynomials are used to model structures, predict stress points, and optimize designs.
• Physics: They help describe motion, forces, and energy in equations of motion.
• Economics: Polynomials are used in cost-benefit analyses and forecasting trends.
• Computer Science: Algorithms often rely on polynomial functions for calculations and simulations.

Understanding standard form polynomial expressions gives you a powerful tool to tackle these real-world challenges.

Tips for Students Struggling with Polynomials

Learning about polynomials can be overwhelming, especially when you're just starting out. Here are some tips to help you master the concept:

• Practice regularly: Like any skill, mastering polynomials takes practice. Solve as many problems as you can.
• Use visual aids: Graphing polynomials can help you visualize their behavior and understand their structure.
• Break it down: Focus on one component at a time—variables, coefficients, exponents, and constants.
• Ask for help: Don't hesitate to reach out to teachers, tutors, or online resources if you're stuck.

Remember, everyone learns at their own pace. Be patient with yourself and celebrate small victories along the way.

Wrapping It Up

In conclusion, mastering the standard form polynomial expression is a valuable skill that opens doors to deeper understanding and practical applications. From organizing terms to solving real-world problems, polynomials play a crucial role in mathematics and beyond.

Here's a quick recap of what we've covered:

• What standard form is and why it matters.
• The components of polynomials and how to convert them to standard form.
• Common mistakes to avoid and practical examples to guide you.
• Real-world applications and tips for students.

Now it's your turn! Take what you've learned and apply it to your studies or projects. And don't forget to share this article with friends who might find it helpful. Together, we can make math less intimidating and more exciting!

So, what are you waiting for? Dive into the world of polynomials and unlock their secrets. Your future self will thank you for it!